Mathematical Constant on:
[Wikipedia]
[Google]
[Amazon]
A mathematical constant is a key number whose value is fixed by an unambiguous definition, often referred to by a symbol (e.g., an alphabet letter), or by mathematicians' names to facilitate using it across multiple
 The imaginary unit or unit imaginary number, denoted as , is a mathematical concept which extends the real number system to the complex number system The imaginary unit's core property is that . The term " imaginary" was coined because there is no ('' real'') number having a negative square.
There are in fact two complex square roots of −1, namely and , just as there are two complex square roots of every other real number (except zero, which has one double square root).
In contexts where the symbol is ambiguous or problematic, or the Greek iota () is sometimes used. This is in particular the case in
The imaginary unit or unit imaginary number, denoted as , is a mathematical concept which extends the real number system to the complex number system The imaginary unit's core property is that . The term " imaginary" was coined because there is no ('' real'') number having a negative square.
There are in fact two complex square roots of −1, namely and , just as there are two complex square roots of every other real number (except zero, which has one double square root).
In contexts where the symbol is ambiguous or problematic, or the Greek iota () is sometimes used. This is in particular the case in
 The
The 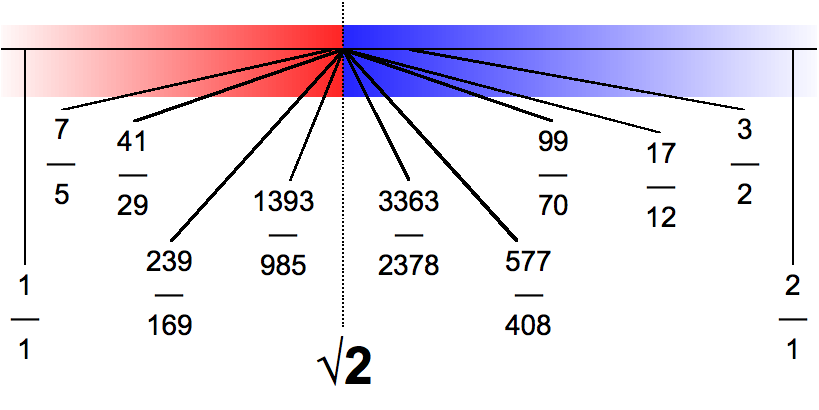 Alternatively, the quick approximation 99/70 (≈ 1.41429) for the square root of two was frequently used before the common use of electronic calculators and
Alternatively, the quick approximation 99/70 (≈ 1.41429) for the square root of two was frequently used before the common use of electronic calculators and
The number , also called the golden ratio, turns up frequently in geometry, particularly in figures with pentagonal
Some constants, such as the
Some constants differ so much from the usual kind that a new notation has been invented to represent them reasonably.
Brun's constant for twin prime
Champernowne constants
cardinal number aleph naught
Sometimes, the symbol representing a constant is a whole word. For example, American mathematician Edward Kasner's 9-year-old nephew coined the names
Constants – from Wolfram MathWorldInverse symbolic calculator (CECM, ISC)
(tells you how a given number can be constructed from mathematical constants)
On-Line Encyclopedia of Integer Sequences (OEIS)Simon Plouffe's inverterSteven Finch's page of mathematical constants
(BROKEN LINK) *Steven R. Finch,
Mathematical Constants
" ''Encyclopedia of mathematics and its applications'', Cambridge University Press (2003).
{{DEFAULTSORT:Mathematical Constant
mathematical problem
A mathematical problem is a problem that can be represented, analyzed, and possibly solved, with the methods of mathematics. This can be a real-world problem, such as computing the orbits of the planets in the solar system, or a problem of a more ...
s. Constants arise in many areas of mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
, with constants such as and occurring in such diverse contexts as geometry, number theory, statistics
Statistics (from German language, German: ''wikt:Statistik#German, Statistik'', "description of a State (polity), state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of ...
, and calculus.
What it means for a constant to arise "naturally", and what makes a constant "interesting", is ultimately a matter of taste, with some mathematical constants being notable more for historical reasons than for their intrinsic mathematical interest. The more popular constants have been studied throughout the ages and computed to many decimal places.
All named mathematical constants are definable numbers, and usually are also computable numbers ( Chaitin's constant being a significant exception).
Basic mathematical constants
These are constants which one is likely to encounter during pre-college education in many countries.Archimedes' constant
The constant (pi) has a naturaldefinition
A definition is a statement of the meaning of a term (a word, phrase, or other set of symbols). Definitions can be classified into two large categories: intensional definitions (which try to give the sense of a term), and extensional definitio ...
in Euclidean geometry as the ratio between the circumference and diameter of a circle. It may be found in many other places in mathematics: for example, the Gaussian integral, the complex roots of unity, and Cauchy distributions in probability. However, its ubiquity is not limited to pure mathematics. It appears in many formulas in physics, and several physical constant
A physical constant, sometimes fundamental physical constant or universal constant, is a physical quantity that is generally believed to be both universal in nature and have constant value in time. It is contrasted with a mathematical constant, ...
s are most naturally defined with or its reciprocal factored out. For example, the ground state wave function of the hydrogen atom is
:
where is the Bohr radius.
is an irrational number and a transcendental number.
The numeric value of is approximately 3.1415926536 . Memorizing increasingly precise digits of is a world record pursuit.
The imaginary unit
electrical engineering
Electrical engineering is an engineering discipline concerned with the study, design, and application of equipment, devices, and systems which use electricity, electronics, and electromagnetism. It emerged as an identifiable occupation in the l ...
and control systems engineering, where the imaginary unit is often denoted by , because is commonly used to denote electric current
An electric current is a stream of charged particles, such as electrons or ions, moving through an electrical conductor or space. It is measured as the net rate of flow of electric charge through a surface or into a control volume. The moving pa ...
.
Euler's number
Euler's number , also known as the exponential growth constant, appears in many areas of mathematics, and one possible definition of it is the value of the following expression: : The constant is intrinsically related to the exponential function . TheSwiss
Swiss may refer to:
* the adjectival form of Switzerland
* Swiss people
Places
* Swiss, Missouri
* Swiss, North Carolina
*Swiss, West Virginia
* Swiss, Wisconsin
Other uses
*Swiss-system tournament, in various games and sports
*Swiss Internation ...
mathematician Jacob Bernoulli discovered that arises in compound interest: If an account starts at $1, and yields interest at annual rate , then as the number of compounding periods per year tends to infinity (a situation known as continuous compounding), the amount of money at the end of the year will approach dollars.
The constant also has applications to probability theory, where it arises in a way not obviously related to exponential growth. As an example, suppose that a slot machine with a one in probability of winning is played times, then for large (e.g., one million), the probability that nothing will be won will tend to as tends to infinity.
Another application of , discovered in part by Jacob Bernoulli along with French
French (french: français(e), link=no) may refer to:
* Something of, from, or related to France
** French language, which originated in France, and its various dialects and accents
** French people, a nation and ethnic group identified with Franc ...
mathematician Pierre Raymond de Montmort, is in the problem of derangements, also known as the ''hat check problem''. Here, guests are invited to a party, and at the door each guest checks his hat with the butler, who then places them into labelled boxes. The butler does not know the name of the guests, and hence must put them into boxes selected at random. The problem of de Montmort is: what is the probability that ''none'' of the hats gets put into the right box. The answer is
:
which, as tends to infinity, approaches .
is an irrational number.
The numeric value of is approximately 2.7182818284 .
Pythagoras' constant
square root of 2
The square root of 2 (approximately 1.4142) is a positive real number that, when multiplied by itself, equals the number 2. It may be written in mathematics as \sqrt or 2^, and is an algebraic number. Technically, it should be called the princip ...
, often known as root 2, radical 2, or Pythagoras' constant, and written as , is the positive algebraic number
An algebraic number is a number that is a root of a non-zero polynomial in one variable with integer (or, equivalently, rational) coefficients. For example, the golden ratio, (1 + \sqrt)/2, is an algebraic number, because it is a root of the po ...
that, when multiplied by itself, gives the number 2. It is more precisely called the principal square root of 2, to distinguish it from the negative number with the same property.
Geometrically the square root of 2 is the length of a diagonal across a square with sides of one unit of length; this follows from the Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite t ...
. It was probably the first number known to be irrational. Its numerical value truncated to 65 decimal places is:
: .
 Alternatively, the quick approximation 99/70 (≈ 1.41429) for the square root of two was frequently used before the common use of electronic calculators and
Alternatively, the quick approximation 99/70 (≈ 1.41429) for the square root of two was frequently used before the common use of electronic calculators and computer
A computer is a machine that can be programmed to Execution (computing), carry out sequences of arithmetic or logical operations (computation) automatically. Modern digital electronic computers can perform generic sets of operations known as C ...
s. Despite having a denominator of only 70, it differs from the correct value by less than 1/10,000 (approx. 7.2 × 10 −5).
Theodorus' constant
The numeric value of is approximately 1.7320508075 .Constants in advanced mathematics
These are constants which are encountered frequently in higher mathematics.The Feigenbaum constants α and δ
Iterations of continuous maps serve as the simplest examples of models for dynamical systems. Named after mathematical physicist Mitchell Feigenbaum, the two Feigenbaum constants appear in such iterative processes: they are mathematical invariants oflogistic map
The logistic map is a polynomial mapping (equivalently, recurrence relation) of degree 2, often referred to as an archetypal example of how complex, chaotic behaviour can arise from very simple non-linear dynamical equations. The map was popular ...
s with quadratic maximum points and their bifurcation diagram
In mathematics, particularly in dynamical systems, a bifurcation diagram shows the values visited or approached asymptotically (fixed points, periodic orbits, or chaotic attractors) of a system as a function of a bifurcation parameter in the syst ...
s. Specifically, the constant α is the ratio between the width of a tine
Tine may refer to:
*Tine (structural), a 'prong' on a fork or similar implement, or any similar structure
*Tine (company), the biggest dairy producer in Norway
* ''Tine'' (film), a 1964 Danish film
*Tine, Iran, a village in Mazandaran Province, Ira ...
and the width of one of its two subtines, and the constant δ is the limiting ratio of each bifurcation interval to the next between every period-doubling bifurcation.
The logistic map is a polynomial mapping, often cited as an archetypal example of how chaotic
Chaotic was originally a Danish trading card game. It expanded to an online game in America which then became a television program based on the game. The program was able to be seen on 4Kids TV (Fox affiliates, nationwide), Jetix, The CW4Kids ...
behaviour can arise from very simple non-linear
In mathematics and science, a nonlinear system is a system in which the change of the output is not proportional to the change of the input. Nonlinear problems are of interest to engineers, biologists, physicists, mathematicians, and many other ...
dynamical equations. The map was popularized in a seminal 1976 paper by the Australian biologist Robert May, in part as a discrete-time demographic model analogous to the logistic equation first created by Pierre François Verhulst. The difference equation is intended to capture the two effects of reproduction and starvation.
The numeric value of α is approximately 2.5029. The numeric value of δ is approximately 4.6692.
Apéry's constant ζ(3)
Apery's constant is the sum of the series Apéry's constant is an irrational number and its numeric value is approximately 1.2020569. Despite being a special value of theRiemann zeta function
The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter (zeta), is a mathematical function of a complex variable defined as \zeta(s) = \sum_^\infty \frac = \frac + \frac + \frac + \cdots for \operatorname(s) > ...
, Apéry's constant arises naturally in a number of physical problems, including in the second- and third-order terms of the electron's gyromagnetic ratio
In physics, the gyromagnetic ratio (also sometimes known as the magnetogyric ratio in other disciplines) of a particle or system is the ratio of its magnetic moment to its angular momentum, and it is often denoted by the symbol , gamma. Its SI u ...
, computed using quantum electrodynamics.
The golden ratio
An explicit formula for the th Fibonacci number involving the golden ratio .
symmetry
Symmetry (from grc, συμμετρία "agreement in dimensions, due proportion, arrangement") in everyday language refers to a sense of harmonious and beautiful proportion and balance. In mathematics, "symmetry" has a more precise definit ...
. Indeed, the length of a regular pentagon
In geometry, a pentagon (from the Greek πέντε ''pente'' meaning ''five'' and γωνία ''gonia'' meaning ''angle'') is any five-sided polygon or 5-gon. The sum of the internal angles in a simple pentagon is 540°.
A pentagon may be simpl ...
's diagonal is times its side. The vertices of a regular icosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrica ...
are those of three mutually orthogonal
In mathematics, orthogonality is the generalization of the geometric notion of ''perpendicularity''.
By extension, orthogonality is also used to refer to the separation of specific features of a system. The term also has specialized meanings in ...
golden rectangles. Also, it appears in the Fibonacci sequence, related to growth by recursion. Kepler proved that it is the limit of the ratio of consecutive Fibonacci numbers. The golden ratio has the slowest convergence of any irrational number. It is, for that reason, one of the worst cases of Lagrange's approximation theorem and it is an extremal case of the Hurwitz inequality for Diophantine approximations. This may be why angles close to the golden ratio often show up in phyllotaxis (the growth of plants). It is approximately equal to 1.6180339887498948482, or, more precisely 2⋅sin(54°) =
The Euler–Mascheroni constant γ
The Euler–Mascheroni constant is defined as the following limit: : The Euler–Mascheroni constant appears in Mertens' third theorem and has relations to thegamma function
In mathematics, the gamma function (represented by , the capital letter gamma from the Greek alphabet) is one commonly used extension of the factorial function to complex numbers. The gamma function is defined for all complex numbers except ...
, the zeta function and many different integrals and series.
It is yet unknown whether is rational or not.
The numeric value of is approximately 0.57721.
Conway's constant ''λ''
Conway's constant is the invariant growth rate of allderived string
Derive may refer to:
* Derive (computer algebra system), a commercial system made by Texas Instruments
* ''Dérive'' (magazine), an Austrian science magazine on urbanism
*Dérive, a psychogeographical concept
See also
*
*Derivation (disambiguatio ...
s similar to the look-and-say sequence (except for one trivial one).
It is given by the unique positive real root of a polynomial of degree 71 with integer coefficients.
The value of ''λ'' is approximately 1.30357.
Khinchin's constant ''K''
If a real number ''r'' is written as a simple continued fraction: : where ''a''''k'' are natural numbers for all ''k'', then, as the Russian mathematician Aleksandr Khinchin proved in 1934, thelimit
Limit or Limits may refer to:
Arts and media
* ''Limit'' (manga), a manga by Keiko Suenobu
* ''Limit'' (film), a South Korean film
* Limit (music), a way to characterize harmony
* "Limit" (song), a 2016 single by Luna Sea
* "Limits", a 2019 ...
as ''n'' tends to infinity
Infinity is that which is boundless, endless, or larger than any natural number. It is often denoted by the infinity symbol .
Since the time of the ancient Greeks, the philosophical nature of infinity was the subject of many discussions amo ...
of the geometric mean
In mathematics, the geometric mean is a mean or average which indicates a central tendency of a set of numbers by using the product of their values (as opposed to the arithmetic mean which uses their sum). The geometric mean is defined as the ...
: (''a''1''a''2...''a''''n'')1/''n'' exists and is a constant, Khinchin's constant In number theory, Aleksandr Yakovlevich Khinchin proved that for almost all real numbers ''x'', coefficients ''a'i'' of the continued fraction expansion of ''x'' have a finite geometric mean that is independent of the value of ''x'' and is kno ...
, except for a set of measure 0.
The numeric value of ''K'' is approximately 2.6854520010.
The Glaisher–Kinkelin constant ''A''
The Glaisher–Kinkelin constant is defined as thelimit
Limit or Limits may refer to:
Arts and media
* ''Limit'' (manga), a manga by Keiko Suenobu
* ''Limit'' (film), a South Korean film
* Limit (music), a way to characterize harmony
* "Limit" (song), a 2016 single by Luna Sea
* "Limits", a 2019 ...
:
:
It appears in some expressions of the derivative of the Riemann zeta function
The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter (zeta), is a mathematical function of a complex variable defined as \zeta(s) = \sum_^\infty \frac = \frac + \frac + \frac + \cdots for \operatorname(s) > ...
. It has a numerical value of approximately 1.2824271291.
Mathematical curiosities and unspecified constants
Simple representatives of sets of numbers
Liouville's constant
In number theory, a Liouville number is a real number ''x'' with the property that, for every positive integer ''n'', there exists a pair of integers (''p, q'') with ''q'' > 1 such that
:0 1 + \log_2(d) ~) no pair of integers ~(\,p,\,q\,)~ exists ...
is a simple example of a transcendental number.square root of 2
The square root of 2 (approximately 1.4142) is a positive real number that, when multiplied by itself, equals the number 2. It may be written in mathematics as \sqrt or 2^, and is an algebraic number. Technically, it should be called the princip ...
, Liouville's constant
In number theory, a Liouville number is a real number ''x'' with the property that, for every positive integer ''n'', there exists a pair of integers (''p, q'') with ''q'' > 1 such that
:0 1 + \log_2(d) ~) no pair of integers ~(\,p,\,q\,)~ exists ...
and Champernowne constant:
:
are not important mathematical invariants but retain interest being simple representatives of special sets of numbers, the irrational numbers, the transcendental numbers and the normal numbers (in base 10) respectively. The discovery of the irrational numbers is usually attributed to the Pythagorean Hippasus of Metapontum who proved, most likely geometrically, the irrationality of the square root of 2. As for Liouville's constant, named after French
French (french: français(e), link=no) may refer to:
* Something of, from, or related to France
** French language, which originated in France, and its various dialects and accents
** French people, a nation and ethnic group identified with Franc ...
mathematician Joseph Liouville, it was the first number to be proven transcendental.
Chaitin's constant Ω
In the computer science subfield of algorithmic information theory, Chaitin's constant is the real number representing the probability that a randomly chosen Turing machine will halt, formed from a construction due toArgentine
Argentines (mistakenly translated Argentineans in the past; in Spanish (masculine) or (feminine)) are people identified with the country of Argentina. This connection may be residential, legal, historical or cultural. For most Argentines, s ...
- American mathematician and computer scientist
A computer scientist is a person who is trained in the academic study of computer science.
Computer scientists typically work on the theoretical side of computation, as opposed to the hardware side on which computer engineers mainly focus (al ...
Gregory Chaitin. Chaitin's constant, though not being computable, has been proven to be transcendental
Transcendence, transcendent, or transcendental may refer to:
Mathematics
* Transcendental number, a number that is not the root of any polynomial with rational coefficients
* Algebraic element or transcendental element, an element of a field exten ...
and normal. Chaitin's constant is not universal, depending heavily on the numerical encoding used for Turing machines; however, its interesting properties are independent of the encoding.
Unspecified constants
When unspecified, constants indicate classes of similar objects, commonly functions, all equalup to Two Mathematical object, mathematical objects ''a'' and ''b'' are called equal up to an equivalence relation ''R''
* if ''a'' and ''b'' are related by ''R'', that is,
* if ''aRb'' holds, that is,
* if the equivalence classes of ''a'' and ''b'' wi ...
a constant—technically speaking, this may be viewed as 'similarity up to a constant'. Such constants appear frequently when dealing with integrals and differential equations. Though unspecified, they have a specific value, which often is not important.
In integrals
Indefinite integrals are called indefinite because their solutions are only unique up to a constant. For example, when working over the field of real numbers : where ''C'', the constant of integration, is an arbitrary fixed real number. In other words, whatever the value of ''C'', differentiating sin ''x'' + ''C'' with respect to ''x'' always yields cos ''x''.In differential equations
In a similar fashion, constants appear in the solutions to differential equations where not enough initial values or boundary conditions are given. For example, the ordinary differential equation ''y''' = ''y''(''x'') has solution ''Ce''''x'' where ''C'' is an arbitrary constant. When dealing withpartial differential equation
In mathematics, a partial differential equation (PDE) is an equation which imposes relations between the various partial derivatives of a Multivariable calculus, multivariable function.
The function is often thought of as an "unknown" to be sol ...
s, the constants may be functions, constant with respect to some variables (but not necessarily all of them). For example, the PDE
:
has solutions ''f''(''x'',''y'') = ''C''(''y''), where ''C''(''y'') is an arbitrary function in the variable ''y''.
Notation
Representing constants
It is common to express the numerical value of a constant by giving its decimal representation (or just the first few digits of it). For two reasons this representation may cause problems. First, even though rational numbers all have a finite or ever-repeating decimal expansion, irrational numbers don't have such an expression making them impossible to completely describe in this manner. Also, the decimal expansion of a number is not necessarily unique. For example, the two representations 0.999... and 1 are equivalent in the sense that they represent the same number. Calculating digits of the decimal expansion of constants has been a common enterprise for many centuries. For example, German mathematician Ludolph van Ceulen of the 16th century spent a major part of his life calculating the first 35 digits of pi. Using computers andsupercomputer
A supercomputer is a computer with a high level of performance as compared to a general-purpose computer. The performance of a supercomputer is commonly measured in floating-point operations per second ( FLOPS) instead of million instructions ...
s, some of the mathematical constants, including π, ''e'', and the square root of 2, have been computed to more than one hundred billion digits. Fast algorithms have been developed, some of which — as for Apéry's constant — are unexpectedly fast.
Graham's number
Graham's number is an immense number that arose as an upper bound on the answer of a problem in the mathematical field of Ramsey theory. It is much larger than many other large numbers such as Skewes's number and Moser's number, both of which ar ...
defined using Knuth's up-arrow notation.Graham's number
Graham's number is an immense number that arose as an upper bound on the answer of a problem in the mathematical field of Ramsey theory. It is much larger than many other large numbers such as Skewes's number and Moser's number, both of which ar ...
illustrates this as Knuth's up-arrow notation is used.
It may be of interest to represent them using continued fractions to perform various studies, including statistical analysis. Many mathematical constants have an analytic form
In mathematics, a closed-form expression is a mathematical expression that uses a finite number of standard operations. It may contain constants, variables, certain well-known operations (e.g., + − × ÷), and functions (e.g., ''n''th root ...
, that is they can be constructed using well-known operations that lend themselves readily to calculation. Not all constants have known analytic forms, though; Grossman's constant and Foias' constant are examples.
Symbolizing and naming of constants
Symbolizing constants with letters is a frequent means of making the notation more concise. A commonconvention
Convention may refer to:
* Convention (norm), a custom or tradition, a standard of presentation or conduct
** Treaty, an agreement in international law
* Convention (meeting), meeting of a (usually large) group of individuals and/or companies in a ...
, instigated by René Descartes in the 17th century and Leonhard Euler in the 18th century, is to use lower case
Letter case is the distinction between the letters that are in larger uppercase or capitals (or more formally ''majuscule'') and smaller lowercase (or more formally ''minuscule'') in the written representation of certain languages. The writing ...
letters from the beginning of the Latin alphabet or the Greek alphabet when dealing with constants in general.
However, for more important constants, the symbols may be more complex and have an extra letter, an asterisk
The asterisk ( ), from Late Latin , from Ancient Greek , ''asteriskos'', "little star", is a typographical symbol. It is so called because it resembles a conventional image of a heraldic star.
Computer scientists and mathematicians often voc ...
, a number, a lemniscate or use different alphabets such as Hebrew, Cyrillic
, bg, кирилица , mk, кирилица , russian: кириллица , sr, ћирилица, uk, кирилиця
, fam1 = Egyptian hieroglyphs
, fam2 = Proto-Sinaitic
, fam3 = Phoenician
, fam4 = G ...
or Gothic
Gothic or Gothics may refer to:
People and languages
*Goths or Gothic people, the ethnonym of a group of East Germanic tribes
**Gothic language, an extinct East Germanic language spoken by the Goths
**Crimean Gothic, the Gothic language spoken b ...
.
Erdős–Borwein constant The Erdős–Borwein constant is the sum of the Reciprocal (mathematics), reciprocals of the Mersenne prime, Mersenne numbers. It is named after Paul Erdős and Peter Borwein.
By definition it is:
:E=\sum_^\frac\approx1.606695152415291763\dots
Eq ...
Embree–Trefethen constant
In mathematics, the random Fibonacci sequence is a stochastic analogue of the Fibonacci sequence defined by the recurrence relation f_n=f_\pm f_, where the signs + or − are chosen at random with equal probability \tfrac12, independently for d ...
Brun's constant for twin prime
Champernowne constants
cardinal number aleph naught
Examples of different kinds of notation for constants.
googol
A googol is the large number 10100. In decimal notation, it is written as the digit 1 followed by one hundred zeroes: 10,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000, ...
and googolplex.
:
Other names are either related to the meaning of the constant ( universal parabolic constant, twin prime constant
A twin prime is a prime number that is either 2 less or 2 more than another prime number—for example, either member of the twin prime pair (41, 43). In other words, a twin prime is a prime that has a prime gap of two. Sometimes the term ''twin pr ...
, ...) or to a specific person (Sierpiński's constant
Sierpiński's constant is a mathematical constant usually denoted as ''K''. One way of defining it is as the following limit:
:K=\lim_\left sum_^ - \pi\ln n\right/math>
where ''r''2(''k'') is a number of representations of ''k'' as a sum of the ...
, Josephson constant, and so on).
Table of selected mathematical constants
Abbreviations used: : R – Rational number, I – Irrational number (may be algebraic or transcendental), A –Algebraic number
An algebraic number is a number that is a root of a non-zero polynomial in one variable with integer (or, equivalently, rational) coefficients. For example, the golden ratio, (1 + \sqrt)/2, is an algebraic number, because it is a root of the po ...
(irrational), T – Transcendental number
: Gen – General, NuT – Number theory, ChT – Chaos theory
Chaos theory is an interdisciplinary area of scientific study and branch of mathematics focused on underlying patterns and deterministic laws of dynamical systems that are highly sensitive to initial conditions, and were once thought to have co ...
, Com – Combinatorics
Combinatorics is an area of mathematics primarily concerned with counting, both as a means and an end in obtaining results, and certain properties of finite structures. It is closely related to many other areas of mathematics and has many appl ...
, Inf – Information theory
Information theory is the scientific study of the quantification (science), quantification, computer data storage, storage, and telecommunication, communication of information. The field was originally established by the works of Harry Nyquist a ...
, Ana – Mathematical analysis
See also
* Invariant (mathematics) * List of mathematical symbols * List of numbers *Physical constant
A physical constant, sometimes fundamental physical constant or universal constant, is a physical quantity that is generally believed to be both universal in nature and have constant value in time. It is contrasted with a mathematical constant, ...
Notes
External links
Constants – from Wolfram MathWorld
(tells you how a given number can be constructed from mathematical constants)
On-Line Encyclopedia of Integer Sequences (OEIS)
(BROKEN LINK) *Steven R. Finch,
Mathematical Constants
" ''Encyclopedia of mathematics and its applications'', Cambridge University Press (2003).
{{DEFAULTSORT:Mathematical Constant
Constants
Constant or The Constant may refer to:
Mathematics
* Constant (mathematics), a non-varying value
* Mathematical constant, a special number that arises naturally in mathematics, such as or
Other concepts
* Control variable or scientific const ...